how to tell what speed a hydraulic pump runs at

Low- and high-speed tests evidence that pump and motor performance can vary widely, depending on the speed and pressure. That'due south critically important in electro-hydraulic actuators and electrical-motor drives.

Contributed by Peter Achten and Robin Mommers, Innas BV, Breda, Kingdom of the netherlands

Hydraulic component manufacturers generally publish pump and motor data that reverberate optimal performance under ideal operating conditions. But experienced pattern engineers know that it is extremely difficult to obtain efficiency or performance data for less-than-perfect, real-world atmospheric condition. Information technology fifty-fifty gets harder when looking at new concepts, such equally electrohydraulic actuators, which run at very low also as high speeds. There are almost no data for these applications.

Innas engineers tested a number of hydraulic pumps and motors at both low and high speeds, with varying results.

To address this information void, Innas recently tested and benchmarked the performance of a number of dissimilar pumps and motors running over a range of pressures and flows. We specifically targeted the types of pumps being considered for electrohydraulic actuators designed for implement systems. Moog, for case, is advocating their radial-piston pump, whereas Rexroth is focusing on axial-piston pumps. Others are looking at gear pumps, both internal and external.

Considering an EHA is constantly moving back and forth, and a lot of the functioning is at low speeds or near standstill, we developed a examination bench that tin can measure at loftier too equally very low operating speeds. Furthermore, we wanted to know the extent of internal leakage for each unit, since meaning leakage will result in a large expressionless-ring. And we wanted to learn the upper speed limits, every bit electric motor developers adopt speeds of v,000 rpm or college, if possible.

Our published written report shows that current pumps and motors are not made for electrohydraulic actuators. They are built for more than or less constant-speed operation, driven by a diesel engine or an electric motor, generally running at 1,500 to 1,800 rpm.

Overview of the specifications of the tested pumps and motors, where z is the number of pistons or teeth, Vg the deportation book, and Vr the volume ratio.

Granted, many of the units tested are specified to not operate beneath a sure minimum speed simply because of significant article of clothing or excessive internal leakage. Nonetheless, these limitations severely hinder their awarding in EHAs. Both expressionless-band and astringent friction volition inhibit the precise operation of hydraulic cylinders, especially considering controllability will go much more of import in the hereafter. For this reason, we accept tested these machines over a broad range of operating conditions.

Results indicate that at that place is a dire demand for the development of new concepts. The Innas floating-loving cup principle represents i such advancement. Its mirrored design with 24 pistons, very curt strokes, a small-scale displacement bending and hydrostatic lubrication provides extremely high efficiency even at start-up. And information technology tin can precisely control significant loads at speeds of just 2 to three rpm with nigh no wear. Only we strongly believe that more breakthroughs are required. We programme to continue to measure the operation of other pumps and motors, and publish updated results on our web site.

Testing groundwork
The study contains results for performance measurements on eight different hydrostatic devices: iii piston pumps, three piston motors, and ii gear pumps. Of import specifications are shown in the accompanying table. The piston-type units have from 7 to 24 pistons. From a physical betoken of view, the number of pistons is like to the number of teeth in a gear pump. Derived geometric deportation volumes range from 23.seven to 32.7 cc/rev.

Comparison the measured power loss at 200 bar for all the devices.

Each pump and motor was measured using a unique exam bench, developed by Innas engineers, which can operate a hydrostatic device at speeds ranging from 5,000 rpm to less than 0.01 rpm. More details are available in the sidebar, "Novel test rig is incredibly versatile."

During tests, sensors in the mechanical components and hydraulic excursion measured:

• Torque on the master shaft, T
• Speed of the main shaft, ω
• Pressure level on the low- and high-pressure side, and in the housing
• Oil temperature at the low- and high-force per unit area side, and leakage
• Main flow rate at the high-pressure side, Q₂
• Leakage menses rate, Q₃

Efficiency and losses
To clarify the different devices, we looked at the measurement results. The 3 well-nigh important quantities used to describe the functioning of the test subjects are the overall efficiency η_t, torque loss T_loss, and leakage Q₃. Efficiency and torque loss are derived from the measured data, while leakage is measured directly.

These graphs compare the normalized torque losses at 200 bar. Linear scale is on the left, log scale on the correct.

It is important to notation that several definitions used here differ from the current standard for measuring the performance of hydrostatic machines equally described in ISO 4409:2019 and ISO 4391 — in item the compressibility correction factors a_ane and a₂. A large group of experts from around the globe hold with the need for these new efficiency definitions.

Overall efficiency. To determine the efficiency of a hydrostatic machine, we first calculated the mechanical power P_yard and hydraulic power P_h:

P_m = Tω
P_h = p₂Q_iia₂ − p_iQ₁

T and ω are the measured torque and shaft speed, p₁ and Q_i are the force per unit area and flow rate of oil through line i (i = low pressure line, 2 = high pressure line), and a_ii is a correction gene to account for the compressibility of oil at loftier pressures. As derived, a₂ = 1 + (p₂/2K_s) where Grand_s is the oil isentropic bulk modulus.

In a pump, mechanical ability converts into hydraulic power, while a motor works the other style around. Overall efficiency η_t is the ratio at which power is converted; for a pump:

η_tP = P_h/P_m = (p₂Q₂a₂ − p_iQ₁)/Tω

and for a motor:

η_tM = P_thousand/P_h = Tω/(p₂Q₂a₂ − p₁Q_i).

Torque loss. Torque losses during operation are calculated by comparison measured torque T to theoretical torque T_th:

T_Ploss = T − T_th
T_Mloss = T_th – T.

The difference in sign is similar to the departure in sign for the efficiencies: torque drives a pump to generate force per unit area and flow, while pressure and flow drives a motor to generate torque. The theoretical torque will thus be lower than the measured torque for pumps, to overcome friction and force per unit area ripples, and the opposite for motors. The theoretical torque tin be calculated using:

T_th = ((p₂ − p₁)V_yard/2π)a_ane

with V₁₀₀₀ the derived geometric displacement book, and a₁ a correction cistron to account for the compressibility of oil at loftier operating pressures.

a_one = 1 − (0.5 + V_min/∆Five)(∆p/K_s)

with V_min the dead volume per cylinder, and ∆V the geometric displacement volume per piston. To compare the torque loss of pumps and motors of different sizes, torque loss can be normalized. The normalized torque loss T_Nloss is defined as one − T_thursday/T for a pump and 1 – T/T_thursday for a motor.

Oil model. Correction factors a_one and a_two used an isentropic bulk modulus K_southward = 1.76 × ten⁹ Pa, which was suitable for our measurements using a Crush Tellus46 oil at roughly 50°C and pressures ranging to 400 bar. Detailed oil modeling concluded that using a single constant majority modulus sufficed in deriving the performance of the machines tested. More details tin be plant in the report, "Performance of Hydrostatic Machines," bachelor at innas.com.

Measurement results
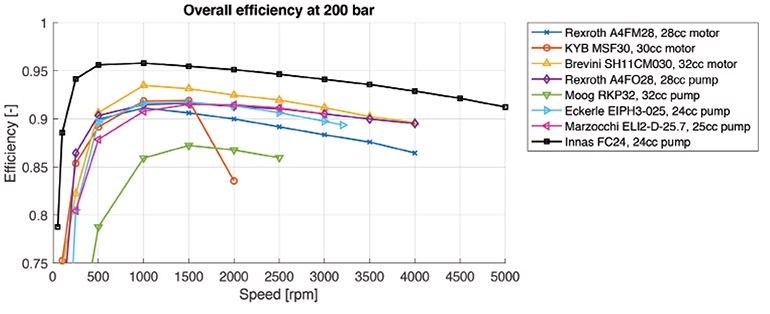
Overall efficiencies of the different pumps and motors were adamant based on the above equations. The results for various shaft speeds at an operating pressure level of 200 bar are shown in the Overall Efficiency graph. All of the tested subjects followed the same trend: Efficiency is low at low operating speeds; increases chop-chop until maximum efficiency is reached somewhere betwixt 1,000 and two,000 rpm; and as shaft speed increases further, efficiency decreases, only at a much lower charge per unit.

At this pressure, the highest summit efficiency of about 0.96 was realized by the Innas pump at one,000 rpm, while the Moog pump had the everyman height efficiency of 0.87 at ane,500 rpm. Ane outlier is the KYB motor's measurement point at ii,000 rpm. Hither, efficiency decreases much faster than was expected. All the same, the unit of measurement was running nigh its maximum rated operating speed, which causes additional torque loss and leakage.

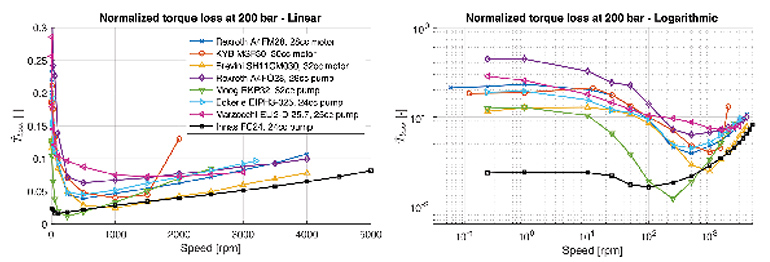
We then used the torque-loss equations to calculate the deviation between the theoretical and measured torque for each device. To properly compare machines of different sizes, these losses have been normalized. The Normalized Torque Loss graphs testify both linear and logarithmic scales at 200 bar. The linear plot shows losses at normal operating conditions, while the logarithmic plot provides some insight into the losses during low speed performance — for example, startup weather condition.

Overall, the torque loss shows a similar trend for motors and pumps. Below 1 rpm (meet logarithmic plot) losses appear to be constant. At some point, the torque loss quickly decreases for increasing shaft speeds, until reaching a minimum. From this speed onwards, the torque loss increases once again, but at a slower rate. This tendency is very much in accordance with the Stribeck curve, and the iii sections draw the transition from coulomb friction (purlieus lubrication), to mixed friction, and viscous friction (hydrodynamic lubrication), respectively.

A comparison between the measured leakage flow rates at higher speeds.

In the linear plot, nosotros see that at this force per unit area level the three motors have similar torque losses at normal operating speeds. Furthermore, the logarithmic plot shows that the Brevini motor outperforms the other ii motors in the low speed range, where it has less torque loss than the KYB and Rexroth motors.

The five pumps show larger differences with respect to each other. Overall, the Innas pump has the lowest torque loss at this pressure, particularly in the depression speed range. This can be explained by the fact that this is a floating-cup type pump, which has almost no contact between the piston and diameter, and thus very picayune coulomb friction. In the viscous-friction section, torque losses in the Rexroth, Eckerle, and Innas pumps increase at roughly the same rate. Torque loss for the Moog pump increases at a faster charge per unit, while the torque loss of the Marzocchi seems relatively constant at higher operating speeds.

The graphs prove that torque loss is more or less abiding at operating speeds below 1 rpm, due to coulomb friction. During the low-speed measurements, each unit tin can be tested during both pump and motor performance. (Because the Eckerle pump is an internal gear pump, it cannot be tested as a motor.) For nearly piston machines the torque loss due to coulomb friction is larger when it is driven as a pump. The exception beingness the Brevini, which has more or less the aforementioned torque loss for both directions.

This tin be explained by the direction in which the piston moves during the high pressure level stroke. When operated as a pump, the piston pushes the barrel to the portplate during the loftier pressure stroke, increasing the friction between those two surfaces and, therefore, the torque loss. When operated equally a motor, the piston pulls the barrel away from the portplate, decreasing friction.

The highest torque loss was plant on the Rexroth pump, which loses up to 60% of torque at these operating speeds. It is worth noting that the two Rexroth units are, apart from silencing grooves in the portplates, the aforementioned product. This was confirmed past comparison the torque losses when they were driven in the same direction.

The normalized torque loss of the Innas pump during low speed motor operation becomes less than nil. In other words, more torque was delivered to the shaft than the theoretical maximum. At these low speeds, leakage volition be larger than the corporeality of displaced oil. In this case, an additional pump provides the right pressure level level. Unfortunately, the calculations no longer apply in this situation, making it incommunicable to derive the overall efficiency. This approach emulates the behavior of units as office of a larger hydraulic network, which is often the instance. It is currently hypothesized that the pressure delivered past this supply pump affects torque on the test specimen'due south shaft as well. Farther research will exist needed to determine why this is the example, and what information technology ways for farther analyses.

Results as well bear witness that at these low speeds there is a significant variation in torque loss at different angular shaft positions. In the case of pumps, this normalized torque is larger than 1, meaning that a certain amount of boosted torque is needed to overcome the friction. Some of the tested specimens needed as much as 80% extra torque to operate at this speed. In the case of motors, the normalized torque is less than 1, meaning that less torque is provided by the supplied oil. One unit showed a torque loss as much as 30%.

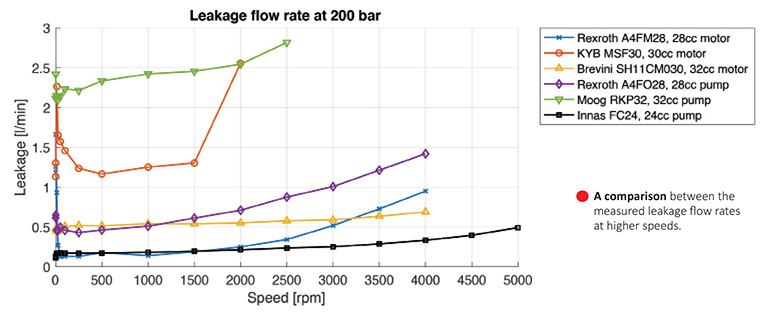
Leakage catamenia rate
The leakage menses rate from the rotary group to the pump or motor housing is measured directly in the examination bench. (Note that the gear pumps do not have an external leakage port, then leakage cannot exist measured.) Internal leakage affects the total output period charge per unit and, thus, the efficiency calculations. Measured leakage for each device at 200 bar showed that for most, leakage increases slightly for increasing shaft speeds. This makes sense, considering increasing the shaft speed will linearly increase the amount of displaced oil. However, the relatively constant leakage menstruum rates at low operating speeds suggest that it does not but depend on shaft speed. A abiding leak catamenia generally ways that the gap through which oil leaks does not change much, indicating that this is probably caused by the precision with which the parts have been made and how accurately they fit together.

This graph shows average leakage flow rates during measurements at less than ane rpm, for units driven both as a motor (left side) and every bit a pump (right side). The width at each pressure level indicates the range of leakage measurements.

Similar to the low speed torque losses, the Low Speed Leakage Flow Charge per unit graph shows average leakage atmospheric condition for measurements at speeds less than 1 rpm. Tests showed a sizeable variation in leakage between the different measurements. Main factors for these larger ranges seem to exist the oil temperature (which is difficult to control during depression speed measurements) and the example force per unit area (mainly for the Moog pump).

Even though in that location is a pregnant spread in the flow rate measurements at different low speeds, leakage is mostly college during motor functioning for most machines. When operated as a pump, the piston pushes the barrel to the portplate during the loftier pressure stroke, decreasing the size of this leakage path. When operated as a motor, the piston pulls the butt away from the portplate, which allows for more leakage. Exceptions are the Innas pump, which has more than or less equal leakage in both directions; and the Brevini motor, which has more leakage when driven equally a pump than as a motor.

Interestingly, while the two Rexroth units showed similar torque losses, there is a big difference between their leakage flow rates — most likely due to the divergence in portplates. Because these measurements take a long fourth dimension, and only little oil flows through the unit, housing temperature decreases over time. This has a large outcome on the viscosity of the leakage oil, causing oil to flow slower at lower temperatures. When measurements are conducted in succession, the housing and oil demand to be heated between each test run.

Innas
innas.com

Novel examination rig is incredibly versatile

The Innas test demote can operate hydrostatic pumps and motors over an extremely wide speed range — from 5,000 rpm to less than 0.01 rpm — cheers to 2 different types of actuators.

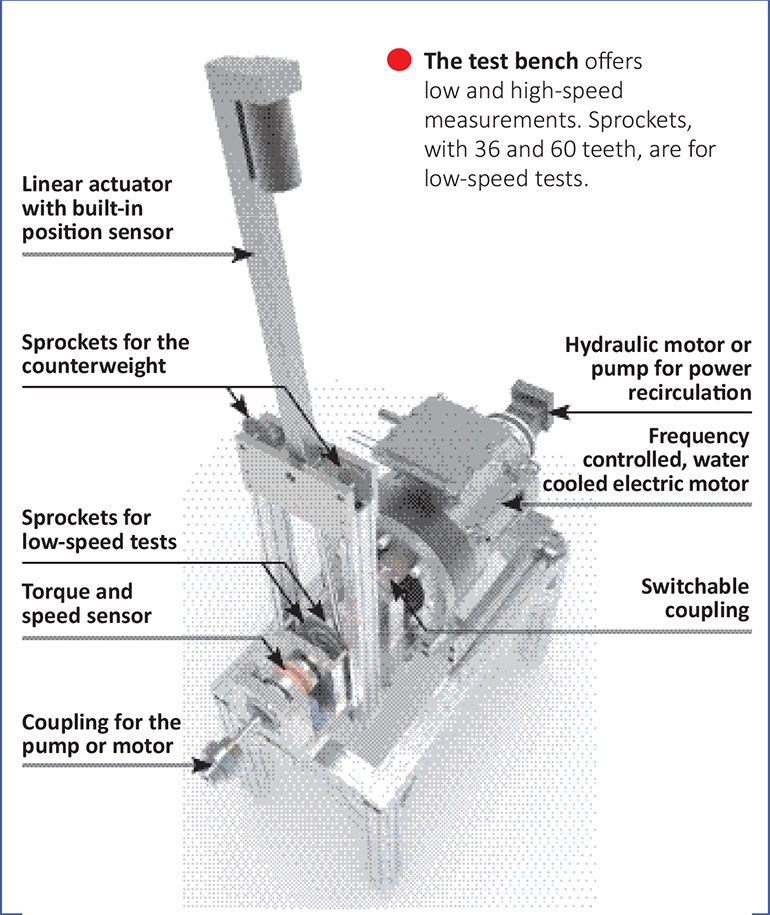The pump or motor tested is coupled to the master shaft, followed by 2 unlike-sized sprockets. An electric linear actuator tin can connect with either sprocket to drive the shaft at speeds from around ane rpm to less than 0.01 rpm. A coupling next to the sprockets lets technicians switch to functioning by a big electric motor. This electrical motor tin rotate the test subject at any speed betwixt 10 and v,000 rpm. A second hydrostatic unit tin can be attached at the back end to recirculate the generated ability. It operates as a pump when the test involves a motor, and vice versa.

Earlier each measurement, the exam subject is driven at a moderate speed and pressure level, to allow information technology and the oil to warm up. When both are at a steady temperature (around 50°C), exam parameters are measured during operation at a number of predetermined speeds and force per unit area levels. This procedure is in accordance with ISO4409:2019 and is used to make up one's mind the geometrical displacement.

After loftier-speed performance tests, depression-speed tests are performed by shifting the coupling and connecting the linear actuator. The integrated prepare-upwardly ensures that the test object, all sensors and hydraulic lines remain on the test demote. Additionally, the oil and pump or motor remain at warm operating temperatures. During depression-speed measurements, the linear actuator first makes a downward stroke followed by an upward stroke. Thus, the unit of measurement is tested both equally a motor and as a pump.

You may also like:

phillipskness1999.blogspot.com

Source: https://www.mobilehydraulictips.com/rating-the-real-performance-of-hydraulic-pumps/

Share this post